Kippenberg, Tobias
Date: Thursday, April 22, 2021
Time: 10:00
Place: scheduled Zoom meeting
Host: Klaus Ensslin
Photonic Chip based Frequency Combs
Tobias Kippenberg
EPF Lausanne
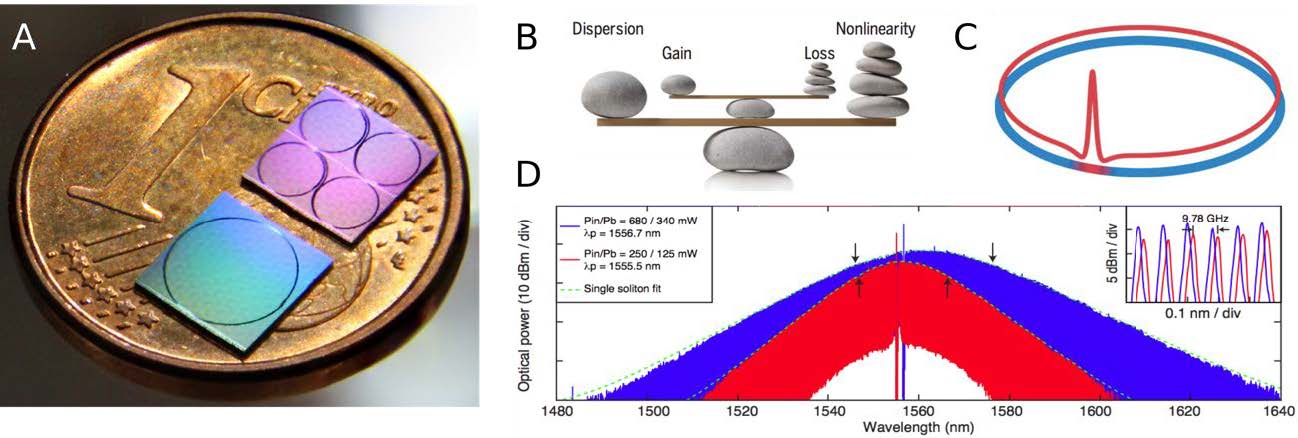
The development of optical frequency combs1, and notably self-referencing, has revolutionized precision measurements over the past decade, and enabled counting of the cycles of light. Frequency combs, have enabled dramatic advances in timekeeping, metrology and spectroscopy. In 2007, it was discovered that such combs can also be generated using an optical microresonator2 using parametric frequency conversion. Importantly, such Kerr combs also enable to generate dissipative temporal solitons (DKS)3,4, which are formally solutions to a driven dissipative nonlinear Schrödinger equation, termed Lugiato-Lefever equation – first derived to describe spatial self-organization phenomena5. DKS have unlocked the full potential of Kerr combs enabling a deterministic route to broadband, and coherent optical frequency combs, whose bandwidth can be enhanced using soliton broadening phenomena, such as Soliton Cherenkov Radiation6. Such Solitons Kerr combs on a chip have enabled to realize counting of the cycles of light, realize dual comb spectrometers on a chip, enabled dual comb based ultrafast ranging7, massively parallel coherent communication8, and offered a novel approach to massively parallel FCMW LiDAR9. Recent advances based on the photonic damascene process10 enable ultra low loss nonlinear photonic circuits based on silicon nitride (Si3N4), have enabled ultra-low losses, and direct integration with on chip pump lasers11.
On the fundamental side, new and theoretically not previously predicted dynamics has been observed ranging from formation of soliton crystals12, soliton switching13, and new type of breather solitons14, and emergent nonlinear dynamics in arrays of coupled resonators15.
Soliton Kerr frequency combs thereby are providing a highly fruitful new playground for fundamental nonlinear science and applications alike.
1. Udem, T., Holzwarth, R. & Hänsch, T. W. Optical frequency metrology. Nature 416, 233–237 (2002).
2. Del’Haye, P. et al. Optical frequency comb generation from a monolithic microresonator. Nature 450, 1214–1217 (2007).
3. Herr, T. et al. Temporal solitons in optical microresonators. Nat Photonics 8, 145–152 (2014).
4. Kippenberg, T. J., Gaeta, A. L., Lipson, M. & Gorodetsky, M. L. Dissipative Kerr solitons in optical microresonators. Science 361, eaan8083 (2018).
5. Lugiato, L. A. & Lefever, R. Spatial Dissipative Structures in Passive Optical Systems. Phys. Rev. Lett. 58, 2209–2211 (1987).
6. Brasch, V. et al. Photonic chip–based optical frequency comb using soliton Cherenkov radiation. Science aad4811 (2015) doi:10.1126/science.aad4811.
7. Trocha, P. et al. Ultrafast optical ranging using microresonator soliton frequency combs. Science 359, 887–891 (2018).
8. Marin-Palomo, P. et al. Microresonator-based solitons for massively parallel coherent optical communications. Nature 546, 274–279 (2017).
9. Riemensberger, J. et al. Massively parallel coherent laser ranging using a soliton microcomb. Nature 581, 164–170 (2020).
10. Pfeiffer, M. H. P. et al. Photonic Damascene process for integrated high-Q microresonator based nonlinear photonics. Optica 3, 20 (2016).
11. Raja, A. S. et al. Electrically pumped photonic integrated soliton microcomb. Nat. Commun. 10, (2019).
12. Cole, D. C., Lamb, E. S., Del’Haye, P., Diddams, S. A. & Papp, S. B. Soliton crystals in Kerr resonators. ArXiv161000080 Phys. (2016).
13. Guo, H. et al. Universal dynamics and deterministic switching of dissipative Kerr solitons in optical microresonators. Nat. Phys. advance online publication, (2016).
14. Guo, H. et al. Inter-mode breather solitons in optical microresonators. ArXiv170505003 Phys. (2017).
15. Tikan, A. et al. Emergent Nonlinear Phenomena in a Driven Dissipative Photonic Dimer. ArXiv200506470 Nlin Physicsphysics (2020).